Summary of Surface Blasting and Damages with Analysis of Two Mitigation Techniques – Presplit and Smooth Blasting
Contents [show]
Introduction
Explosives have been used by humans as an effective tool since ancient times, typically in the art of war. Blasting, however, utilizes explosives in a constructive way by focusing the vast energy produced by the explosion towards breaking rock masses into more manageable fragment sizes, while simultaneously mitigating the damages caused by the explosions to other structures or permanent rock masses. Rock blasting has been used in mining for hundreds of years and continues up to this day, but other uses, like excavation of rock slopes for highways through mountains or the preparation of rock for the foundations of buildings, has grown considerably within the last century.
As blasting has become more extensive, the damages caused have grown as well. For the extent of this paper, the discussion of damages will be limited to a general sense and two specific case histories, the Ekati Mine in Canada and the excavation of high rock slopes in China. Damages can be considered loss of strength to the remaining rock mass in which the blasting occurs. Like many procedures that are centuries old, blasting was once simply done by intuition and experience. As the use of blasting progressed, however, how to blast became better documented in manuals and textbooks to move blasting away from rules of thumb into a more scientific method, and with this progression, ways to limit the extent of damage by using certain techniques, such as presplit and smooth blasting.
Understanding of the damages resulting from blasting must stem from knowledge of blasting itself. Therefore, preceding the discussion on blast mitigation is a general overview of surface blasting. The mechanics of rock blasting and the uses of blasting, some of which were listed previously, will be discussed. Additionally, the typical terms and components of a blast will be outlined. Finally, the damages associated with blasting will be summarized before the specific case histories are evaluated.
Mechanics of Rock Blasting
The basic principle behind rock blasting is the release of energy from a chemical compound, the explosive, in the form of expansive gases and heat inside a hole drilled into a relatively concentrated part of the rock mass. This process is fairly complicated but can be broken into three main components: initial pressure buildup, wave transmission and air blast (USACE 1972). The rapid expansions of gases in the hole can create pressures reaching a maximum of 100,000 atmospheres and occurs within milliseconds (USACE 1972).
The immediate area surrounding the drilled hole undergoes crushing from the initial release of gases to a distance approximately equal to the initial radius of the drill hole (Langefors 1978). In this vicinity, the compressive forces from the gas pressure are larger than the compressive strength of the material, thus crushing. However, beyond this area, compressive forces are reduced below the compressive strength of the rock. The crushed area is marked in Figure 2.1, taken from the USACE (1972) blasting manual.
Despite the fact that the wave no longer results in failure of the rock through direct compression, the rock will still fracture. This failure type can be seen as the radial cracks extending in Figure 2.1 perpendicular to the circumference of the drill hole. As the compressive wave moves outward in a concentric ring, hoop stresses, as termed by the USACE (1972) manual, or tangential stresses, as Langefors (1978) refers to them, create tensile stress on the rock, noted as σT in Figure 2.1. Unconfined tensile strength of a rock is much lower than that of compression. Since the wave can maintain the minimum intensity needed for tension over a greater distance, even as the intensity falls well below that needed for crushing, the radial cracks extend farther than the crushed zone. To gauge the order of magnitude of this distance, a blast hole with a diameter of 40mm can have a range from tens of centimeters to a meter for radial cracking (Langefors 1978).
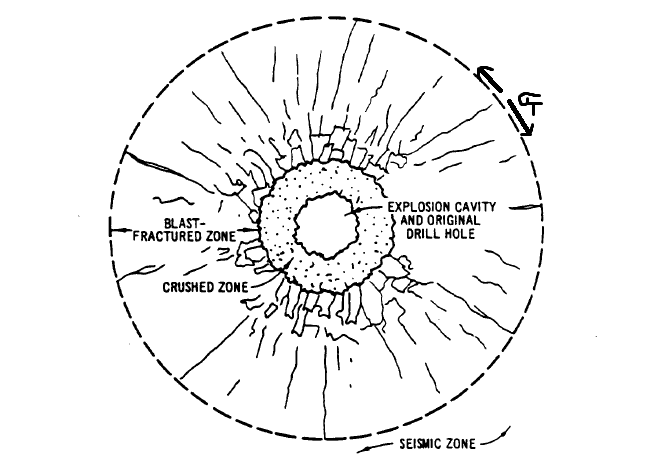 Figure 2.1: Zones of Fracturing and Deformation. Adapted from USACE (1972)
Figure 2.1: Zones of Fracturing and Deformation. Adapted from USACE (1972)
Another failure mode present in a blast is the rebounding of the compression wave upon hitting a free face. As the wave hits a free face it is rebounded back, reversing the direction of the wave while the tail end of the same wave is still pushing forward, putting the rock in tension (USACE 1972). This creates spalling upon the free face. Free faces can be any pre-existing joint or bedding plane within the rock mass, fractures created by the neighboring blast, or the opening face. The concept of rebound is important when considering blast damages because it can be used as a means to reduce any further fracturing to a final face, which will be covered in greater detail later. Although fracturing and crushing are limited to the zones above, the shockwave produced by the explosion propagates outward in the form of primary waves and shear waves for thousands of feet.
Finally, the large build-up of gases must be expelled in what is the slowest of events to take place during a blast. The previous processes created the conditions needed for the expanding gases to actually break the rock (Langefors 1978). The expelling of the gases pushes the free face outward and expands the radial cracks, creating tension and further failure. If the blast does as intended, the cracks will extend to the free face and the burden will be propelled outwards completely. Ensuring that this entire process occurs is heavily dependent upon the spacing of the blast holes, bench height, and the thickness of the burden, among other factors. These will be covered further in Section 4.
Types of Blasting
Blasting can be separated into two general categories, surface blasting and underground blasting. Both types share the same principles, turning an unmanageable rock mass into smaller, broken pieces. Surface blasting will be the focus and is useful for removing overlying material for mines or cutting slopes for highways. It can also be used in obtaining the required depth for placing a foundation. For excavation, the material only has to be fragmented to a degree easy for removal, and uniform fragments are not really required. In comparison, production blasting in quarries must create uniform fragmentation so the material can be used as rip rap or aggregate.
Regardless of the type, blasting poses problems to the engineer. Certain problems, like airblast, the excessive noise resulting from an explosion, or fly rock, material being thrown from a blast, are more relevant to urban environments where blast sites are surrounded by existing buildings and pedestrians. While fly rock and airblast are a concern for any blast, the extent of damage from vibrations, gas pressures, and poor blasting are summarized for the two case studies. In these instances, the excavation of material for a permanent high rock slope and production blasting for mining are analyzed for the extent of blasting damage.
Typical Components and Terms of Blasting
Approximately eight parameters can be used to describe most blasts, despite the location or use of the blast. These are listed in Table 4.1. For the scope of this paper, each is only delved into enough as to give the reader basic understanding, and therefore, some aspects of blasting are left out altogether. Most of the parameters are interdependent on one another and changing one will affect the magnitude of the others.
| Table 4.1: Parameters of Blasting |
| 1. Explosives |
| 2. Blast Hole Diameter and Drilling |
| 3. Burden |
| 4. Bench Height |
| 5. Spacing |
| 6. Detonation |
| 7. Stemming |
| 8. Properties of the Rock Mass |
Explosives
The term explosive refers to a “chemical compound or mixture of compounds that undergoes a very rapid decomposition when initiated by energy in the form of heat, impact, friction, or shock.” (Dick 1982). A typical law of nature is that unstable substances want to become more stable, and as such, the explosives will become so by converting into a very hot gas and releasing large amounts of energy, which causes the immense pressures and the associated shockwaves responsible for the failure mechanisms of rock.
A list of different explosives and some of their properties and ingredient compositions can be found in Figure 4.1 (USACE 1972). Two types of explosives can be used, low explosives and high explosives, which are defined by the velocity in which the chemical reaction takes place. For a low explosive, the reaction moves below the speed of sound, termed deflagration, while a high explosive’s reaction occurs faster than the speed of sound, called detonation (Dick 1982). An example of a low explosive is black powder and is the main low explosive used commercially although not very effective at fragmenting rock (Dick 1982); all other explosives used in commercial blasting are normally high explosives. As such, detonation velocity is one of the most important characteristics in classifying an explosive.
Figure 4.1: Explosive Ingredients and Properties. USACE (1972)
Detonation velocity is a function of the density of the explosive, as shown in Figure 4.1, the less dense an explosive, the lower the detonation velocity. Density serves another purpose; which is to determine the amount of charge placed in a hole. Typically, the amount is given in weight per length, and this varies according to the density of each explosive and how that explosive is packed in the hole. Density and water resistance are important, too, when a high water table is expected or blasting underwater is needed. If the explosive is less dense than water it will not sink into the blast hole, and an explosive that is not resistant to water cannot be used at all.
Another classification of explosives used is strength, and this is typically measured based on weight or volume. However, it does not give a good measurement of the ability of the charge to do work and can be misleading (USACE 1972). Another important property that should be considered when blasting in enclosed spaces, like a tunnel, is fume class, which classifies explosives based on if poisonous gases are released. Explosives classifying as one that gives off poisonous gases should not be used in tunnels but typically pose little concern to open-surface blasting.
A general formula provided by Langefors (1978) equates the quantity of charge needed to break the burden as a function of geometry, explosive, and the rock parameters. Because these parameters are interdependent, this equation can be rearranged and some variables held as constant to define other parameters. This is important to remember for each individual term following and in a design.
Q = f(V, K, E, h, d, s, p, u, ci)
Where: V= burden, K= bench height, E=spacing between blast holes, h= height of the charge, d= hole diameter, s= weight strength, p= density of explosive, u=detonation velocity, ci= rock characterization
Blast Hole Diameter and Drilling
The blast hole diameter is the leading factor in determining other parameters, mostly because burden is a direct function of the diameter, and then other parameters are determined from the burden (Dick 1982). With that said, one should note that if two moderately differing diameters are loaded with the same quantity of explosive in the hole’s bottom or with an equal, uniform charge per length of the hole, then little variation is noted in breaking force between them (Langefors 1978). While this can seem counterintuitive to the previous statement, the change in diameter size is still relevant.
What a change in diameter allows for is a greater concentration by weight of charge at the bottom of the hole or per length, therefore increasing the size of the burden that can be blasted. Thus, as diameter increases, burden increases and so does the maximum height of the bench. Inversely, as the diameter increases, the cost decreases to a level because it is cheaper per unit volume to drill and cheaper, bulk explosives can be used (Dick 1982). This is limited, though, because large diameter holes will have fragmented rock that is more expensive to remove, and a compromise must be reached optimizing the benefits of large and small diameters. In addition, the size of the hole drilled is dependent upon factors such as site conditions, drill rigs and labor available, costs, and rock type (USACE 1972). These limitations are what keep contractors from simply drilling the larger diameter holes and blasting the larger amounts of rock, which is typical of mining because they have the ability to drill large diameters and remove the coarser fragments.
Drilling can be accomplished by percussive drills, rotary drills, and various other procedures which in turn can be performed by varying drill bits and drill rigs, each with their own advantages and disadvantages. Regardless of type and technique, the drilling accuracy is what distinguishes theory from actuality because these deviations can result in variations of burden as seen in Figure 4.2 (Langefors 1978). Depending on the deviation, the burden can become too thin or too thick at the toe, creating the unintended new free face represented by the dotted line in Figure 4.2. This issue compounds with multiple rows, as the effective burden of the next row increases or decreases. Section 4.3 describes the issues related to overly thick or too thin burden lengths.
The angle at which a hole is drilled will also affect burden dimensions, and drilling parallel to a sloped free face is often done to reduce unevenness of burden at the top and toe of the free face experienced when the drilling is vertical. Additionally, the borehole is drilled below the intended final floor elevation. This is termed the subdrill depth and helps to pull all the rock from the floor. The subdrill depth ranges from from about 0.2 to 0.3 times the burden, seen as J in Figure 4.3. This depth is reduced if angled drilling is used as a result of the burden at the toe being more easily removed (USACE 1972).

Figure 4.2: Result of Deviated Drill holes. Langefors (1978)
Burden
The burden can be defined as the distance between the drill hole and the free face. In a typical bench blast, Figure 4.3, and a more complicated blast design, Figure 4.4, the burden is labeled as B (Dick 1982). Burden also applies to the distance between two rows of blast holes if multiple rows are detonated in sequence. The burden can be related for bench blasting, in general, directly to the diameter of the blast hole. A very easy rule of thumb, accounting for some drill error, is simply for every inch of diameter, you have 1-meter of burden (Langefors 1978). Thus, if you have a 1-inch diameter hole, your burden is 1-meter; a 2-inch diameter hole gives you 2-meters and so on. While this mixes English units with metric units, it is easily remembered when doing initial calculations for a rough estimate.
If the height of the bench falls below 1.8 * B, then the total burden length allowed becomes a function of diameter and height of the bench, instead of just diameter (Langefors 1978). Decreasing the height while keeping the diameter constant will decrease the burden width. Therefore, it is beneficial to have a bench height above 1.8 * B because more rock can be blasted for each detonation. Other considerations that affect burden length are multiple rows and direction of the free face.
Underestimating or overestimating the burden poses different issues, respectively. Underestimating the burden will create airblast and fly rock. Overestimating the burden, on the other hand, results in blocky fragmentation, issues at the toe, and unnecessary ground vibrations (Dick 1982). As such, a semi Factor of Safety is built into calculations of the burden to reduce the maximum value to an effective value based on typical inaccuracies of drilling (Langefors 1978). No corrections are made for underestimating, but when airblast and fly rock are detrimental, like urban environments, other precautions can be taken.
Figure 4.3: Isometric View of Typical Bench Blasting Diagram. Dick (1982)
Bench Height
As already mentioned, bench height can have significant consequences on the burden thickness. It is measured, simply, as the depth of the drilled hole, identified as H in Figure 4.3. Typical height to burden ratios are between 1.5 to 4.0 (Dick 1982). While this is below the 1.8 recommended by Langefors, a ratio of 1.5 is more easily remembered and variability is expected. Going below a ratio of 1.5 will have the effect of producing excessive airblast and fly rock as well as uneven or poor fragmentation of the burden (Dick 1982). A ratio greater than 4.0 leads to a greater chance of the drill hole at the bottom of the bench height deviating from its intended position, or the explosive charge being cutoff along the length, resulting in unexploded charges (Dick 1982). In situations where location and geometry necessitate an undesirable height, solutions are available to correct for the above disadvantages.
Spacing
In Figure 4.3, the spacing is seen as S between the blast holes for a single row. However, as seen in Figure 4.4, the blast patterns are not always so simple, and for those cases spacing is perpendicular to the burden. Like the previous parameters, typical values are given as a function of other parameters, in this case a ratio of spacing to burden, where a good first estimate is 1.5 (Dick 1982). Spacing is also affected by the timing of detonation, and a single blast can have delays between multiple rows or delays among individual blast holes in a single row. If no delay is used, the simultaneous detonation of a single row allows for a spacing to burden ratio of about 2.0 (Dick 1982). When blasting for a final face, spacing is important because too great a spacing will create a wave pattern along the face due to incomplete fracturing while too small of spacing creates its own issues by cratering and crushing rock between the drill holes (Dick 1982).
Figure 4.4: Plan View of Sapcing and Burden for Different Free Face Orientations. Dick (1982)
Detonation
The process of initiating the main charges begins with an electrical surge sent into a blasting cap. The blasting cap then ignites a detonating cord or the main charge itself. A primer, or catalyst, is another explosive set off by the blasting cap or detonating cord that quickens the reaction and increases the efficiency of the main blast (USACE 1972). Detonation of blasts is measured on the same order of magnitude as the chemical reaction and mechanical fracturing of the rock, typically in milliseconds.
With such a minute time-scale, the sequence in which the charges are detonated affects the overall blast. As was mentioned in spacing, detonation of all charges in a single row at once allows for greater spacing, due to the energy being released simultaneously. However, the use of delays from row to row and between individual blast holes is beneficial because it creates free surfaces for the next charge to take advantage of, will optimize fragmentation, and reduces the ground vibrations. Reduction of ground vibrations is proportional to reduction of damage to the rock mass, as well as reducing damages to surrounding structures.
Stemming
A blast hole is loaded intentionally with space between the top of the charge and the opening of the hole. The opening at the top is called the collar, T in Figure 4.3. The space is then packed with an inert substance to confine the charge below, known as stemming (Dick 1982). The additional confinement focuses the blast energy into the rock mass and in so doing, reduces airblast which, in regards to the surrounding neighbors' perceptions of blasting, is vital because the violent noise from airblast can cause an influx of complaints (Dick 1982). A median must be reached between too little a stemming distance and too great, similar to the other parameters. The result of a stemming too small creates flyrock and airblast, part of the issue with short bench heights, and too large a stemming distance will result in boulders at the top of the burden as well as overconfinement. A rough estimate provided by Dick gives a good initial estimate for stemming distance equal to 0.7 times the burden (Dick 1982).
Properties of the Rock Mass
Properties of the rock mass play a vital role in determining all of the previous parameters. Looking at it from the perspective of the mechanics of a blast, strength and degree of jointing and fracturing affect the way it fails. As discussed earlier, the compressive strength is much greater than the tensile strength, and the ratio of the two is a called the blasting coefficient. Values of the blasting coefficient can be 9 to 15 for quartzite, between 10 and 17 for most igneous rocks like granite and basalt, and range from 17 to 23 for sedimentary rocks such as limestone (USACE 1972). The blasting coefficient is a good indicator of the degree of spalling, with a higher number indicating greater spalling in the rock.
Another parameter linking the properties of the rock mass to blasting is the powder factor, or the weight of explosive in pounds required to blast a cubic yard (USACE 1972). A dense rock requires more explosive to displace the rock, and vice versa. In addition, the hardness of a rock mass is important in determining charge. Placing too great a charge in a very hard rock can result in excessive flyrock and airblast while an over-charged blast hole in soft rock is less likely to have this happen. On the other hand, if a hard rock is under-blasted then removing the resulting material is difficult relative to a soft rock, where removal is likely to still be possible (Dick1982).
Fracturing and jointing of a rock, like other aspects of rock engineering, create planes of weakness for failure to occur. This can be beneficial to a blast if the rock mass is heavily fractured with close-spaced jointing because the development of new fractures is not needed, and a smaller amount of explosive can be used to heave the rock. However, highly jointed rocks can pose an issue for presplitting because the gases will infiltrate the joints and loosen them (USACE 1972). For more widely spaced joints, the fragmentation can become uneven and result in large blocks remaining. Adjusting the spacing is a useful way to limit this. Smaller spacing will increase the chance that individual blocks of rock will be penetrated, and in so doing, form new fractures within the block. Compared to larger spacing, the blocks formed may remain largely intact from no direct contact with the explosives and favoring of the blast energy to escape along the existing joints.
Figure 4.5 from Dick gives a visual representation of how to prevent the explosive force from penetrating the existing weaknesses, resulting in more favorable fragmentation. This is accomplished by a careful borehole log and then stemming where the joints are thought to be (Dick 1982). Again, these joints can be crucial in pre-splitting to prevent an unstable final face. Alternating layers of strong and weak material behave similar to jointing because the energy will want to escape through where it is easiest, leaving the stronger material intact.
Figure 4.5: Stemming of Planes of Weakness. Dick (1982)
Damages and Mitigations
Brief examples have already been given as to what can be considered damage. Damages to the rock mass stem from the mechanics of a blast, mainly the production of waves and the work of expanding gases. Overbreak, excavation stability, and release of load are common types of damage. It should be noted, though, that in the case of mines and other slopes, deep seated failures are not typically attributed to blasting, but shallower failures are of concern (Hoek 2007). In general, the affect blasting can have on the rock mass is shown in the Hoek-Brown strength criteria. Equation 5.1 is the modified Hoek-Brown criterion for rock masses and Equation 5.2 is the equation for the material constant mb, or the constant describing the rock mass (Hoek 2002). Within Equation 5.2 is the parameter D, disturbance factor, which for an undisturbed rock mass is equal to zero, while a bad blast can have a D value of 1, significantly lowering the strength (Hoek 2002). This gives a numerical validation of how blast damage is typically taken into account for slope stability.
Equation 5.1: Hoek-Brown Failure Criterion. Hoek (2002)
Equation 5.2: Material Constant. Hoek (2002)
Overbreak can be described as the spread of the effects of a blast beyond the intended blast zone, or the propagation of cracks into the solid rock remaining. This is limited to approximately the burden distance and one can expect about an 80% reduction in rock strength at this distance (Peterson 2001). A greater distance and extent of damage, though, can be expected if planes of weakness are extensive in the rock mass.
Similar to overbreak is excavation stability. The vibrations coming from a blast can create movement along existing joints, and in so doing, weaken the bonds between rock blocks, up to hundreds and thousands of feet away from the charge (Peterson 2001). Frequency of the wave is also important because a rock mass may experience more movement if joint spacing is equal to frequency. Figure 5.1. provides an example of how waves can create movement along joints with the coupling effect of frequency (Peterson 2001). In addition to vibrations, movement from the expansion of the rock mass from gases and pressure changes are equally likely to affect shear strength along the jointsBy reducing the shear strength of joints from movement and heave, a future excavation can create planar and wedge failures in the rock mass.
Figure 5.1: Wave and Frequency Effect on Joints. Peterson (2001)
Release of load damage is the process of spalling described in the mechanics of a blast. Hoek (2007) provides a great analogy of this mechanism. It is similar to dropping a steel plate on several rubber mats and letting the mats rebound. Upon rebound, the steel plate is thrown upwards and the rubber mats separate as they, too, accelerate upward. This separation is the cause of tension failure in rock. Release of load can form vertical cracks up to 55 meters beyond new faces and can cause instability of the rock face as a result of the cracks (Hoek 2007).
Limitation of these damages is vital to project life spans and safety. The greatest reduction in damage, but by no means the easiest or most effective, would be to mechanically excavate the rock, eliminating the use of explosives altogether. This has been done effectively in underground tunneling for many projects and in surface mining, specifically the Bougainville copper mine in Papua New Guinea (Hoek 2007). Mechanical excavation simply cannot be used all the time because of factors ranging from cost and scale to strength of the intact rock. Therefore, blasting is employed but this comes at the cost of potentially more damage.
Vibrations
The P and S waves generated from the blast propagate outwards until they eventually attenuate below values of any significance. These waves are measured as peak particle velocities per second (PPV) either in mm or feet; the amplitude of the wave depends on two main factors among many, distance from the blast and weight of the explosives. Relations have been made between PPV and strains in rock, and from this, the likelihood of new fractures forming or existing fractures slipping (Peterson 2001). Thus, knowing how much the waves attenuate in rock and at what distance vibrations will be of concern is imperative in being able to gauge damage. Monitoring devices are employed to measure this in real time and allow for calibration of blasting design.
Table 5.1 taken from Peterson (2001) offers a brief guideline as to the ranges vibrations should be kept at for typical mining operations to limit rock damage. Keeping in mind that the amount of explosives detonated is one of two main parameters determining amplitude of vibrations, one can then see the importance of detonation sequence beyond ensuring even fragmentation. A time period of 8-ms or 9-ms between detonations of charges is considered as a typical threshold around which charges are considered separate explosions (Dick 1982). In addition to delay between charges, lower charge weights can be accomplished by smaller diameters and closer spacing, as is the case for blasting a final face. Next, distance is taken into account. The main charges in a blast, as discussed for smooth blasting and presplit blasting later, are drilled at a prescribed distance from the final wall face to allow for a dissipation of wave energy.
Other parameters affecting vibration have already been mentioned in Section 4. Excessive overburden and stemming can cause over-confinement of the charges, redirecting the blast energy into the rock instead of outwards. Besides poor design of the burden, excessive overburden can be a result of not allowing the previous row in multiple-row blasting to clear before detonation of the next row. This can be remedied by greater delay between rows. Similarly, charges placed in deep subdrilling cause over-confinement and will result in excessive vibration (Dick 1982).
| Rock Quality | Threshold Limits |
| Excavation in poor quality rock | 200-600 mm/s (0.66 – 1.97 ft/s) |
| Excavatioon in good quality rock | 600-2000 mm/s (1.97 – 6.56 ft/s) |
| Excavation with unfavorable jointing and potential for unstable blocks along walls | 100 – 600 mm/s (0.33 – 1.96 ft/s) |
Table 5.1: Thresholds of PPV for Rock Quality. Peterson (2001)
Smooth (Cushion) Blasting
By reducing the weight of individual charges and decreasing the spacing for the final face, smooth blasting allows for less damage to the remaining rock. The main objective of smooth blasting is to leave a small berm of rock between the final face and the main blast, and then blast the berm with tightly spaced, lightly loaded blast holes. This forms a continuous crack between holes, giving an evenly contoured final face while keeping large blasts at great enough of a distance to limit damaging vibrations.
In general, a smooth blast will have dimensions with the hole spacing being less than the burden, as mentioned above. The height of a smooth blast is limited by accuracy of drilling, so typically, if a bench is over 60-ft, the blast is separated into two lifts, reducing the overall height and compounding error from drilling (USACE 1972). Cushion blasting is a form of smooth blasting that takes advantage of the effect air space has on reducing overall crack density, thus reducing damage to the remaining rock wall (USACE 1972). For current reference and future reference, Figure 5.2 shows a typical blast design and sequence of smooth blasting and presplit blasting (Hu et al. 2013).
Figure 5.2: Excavation Sequence of Two Methods a) Smooth Blasting and b) Presplit Blasting. Hu et al. (2013)
Presplit Blasting
A similar form of protective blasting is presplit blasting. Presplitting has the intention of forming a free face barrier, between rock to be removed and rock that is too remain, by detonating a row of lightly packed charges prior to the main blasts along the final contour face.For a comparison with regular blasting, Figure 5.3 is a good representation where the presplit face is on the left and the more broken face of regular blasting is on the right (Hoek 2007). In an ideal presplit, a continuous fracture will form between each blast hole creating a final contour while minimal crushing and radial cracks occur, leaving half of the drill hole still visible and very little breaking of the final face, as seen in Figure 5.3.
One common design among many of a presplit row is a spacing of 24-inches center to center with 3-inch diameters (USACE 1972). Height of the drill hole for presplitting is similar to smooth blasting because deviation may result in the holes not forming a continual fracture. A common range for bench height is between 25-feet and 40-feet (USACE 1972).

Figure 5.3: Comparison between Presplit and Regular Blasting. Hoek (2007)
Mechanics of Smooth and Presplit Blasting
In order to better understand how smooth and presplit blasting affect the final face, one needs to know the mechanisms utlized. Some of this was previously explained in the crack formation, but this can be defined further. Model studies have shown that a charge in contact with the wall will create a region of denser cracking versus a charge with space between the wall and the charge. The charge with space will form cracking of lower density but the cracks will have with the same length as the charge in contact with the wall (Langefors 1978). Since only a single crack is needed to extend from one blast hole to the next, obviously creating more cracks than necessary will create a more damaged rock face. Model studies have also shown that overcharging will have a similar effect of forming dense cracking (Langefors 1978). Again, because the objective is a single, continuous crack , the charge should be given space between the wall of the hole and be of smaller size to reduce overall cracking while still creating a continuous fracture.
Aiding in the process of a crack formation from hole to hole is the distribution of stresses. A circular opening in elastic material put under tension will experience the largest stresses at the two points nearest and farthest from the imposed stress (Langefors 1978). These two points guide the direction of the stress and explains why a blast, imposing tensional stress, will have cracks tending in a fairly straight line during presplitting and smooth blasting. Finally, the design also affects how a fracture forms. A ratio of spacing to burden below 0.8 is preferred because the crack formed will be straighter compared to a ratio above 0.8. Delay between blasts is similar to the burden ration in that a shorter delay between blasts will create a more linear fracture line. Simultaneous detonation will create the straightest face, while shot by shot blasting will form an erratic face. Typically a short delay time is used with results somewhere in between simultaneous and shot by shot blasting (Langefors 1978).
Case Studies
Two case studies will be examined, one looking at the use of presplit blasting in a mine and the other comparing presplit blasting with smooth blasting of the final wall faces. The first study was done at the EkatiTM Diamond Mine by Peterson and the second study was done by Hu et al. of high rock slope excavations in China.
Blast Damage Mechanisms at EkatiTM Mine by Peterson (2001)
The EkatiTM Diamond Mine is located in the Northwest Territory, Canada. The study was done to determine the quality of the final pit wall using the presplit blasting technique. As a part of the study, vibration and gas penetrating equipment were set up and a total of 3 blasts were monitored. The blasts monitored included a production blast, a wall control blast, and a pre-shear blast. For each blast, a summary of vibration and gas penetration data along with assessment of the rock mass was carried out.
Beginning with the production blast, the data obtained from this blast can be used as a reference in comparing the other two blasts because no measures, i.e. presplitting, were used to dampen the affects. The monitoring equipment was set up in the rock behind the last row of blast holes and observations were taken of this remaining rock mass, treating it as a hypothetical final face. Figure 6.1 taken from Peterson (2001) is a post-blast cross-section pointing out the extent of damages. Fragmentation was observed 5-meters behind the last row of blast holes and additional cracks were observed in the intact rock up to 25-meters, with dense cracking observed up to 10-meters. The 25-meter cracks were expected to be a result of displacement along joints. Gas pressure data recorded a pressure drop of negative 67 kPa and the vibration data provided results with a peak of 1730 mm/s.
Figure 6.1: Cross Section Showing Varying Degrees of Damage from Production Blasting. Peterson (2001)
The wall control blast was done with a presplit fracture in place prior to the production blast. For data collection and monitoring, two rows of smaller, lighter packed buffer blast holes and then the rows of production blasting were used. Monitoring equipment was placed 5-meters back from the presplit contour. For this blast, damage could be seen in the remaining rock mass, specifically at the corners, and small wedge failures along the crest. The gas pressure data gave a peak value of negative 70kPa and the PPV values measured were considerably lower than the production blast, with one instrument measuring approximately 600 mm/s and the other a little less than 1000 mm/s.
The final blast measurement was done for the actual presplit blast. This blast generated a continuous fracture along the line of blast holes, with some cratering and displacement. Instrumentation was again placed 5-meters behind the presplit row. The peak gas pressure measurement was a positive 67 kPa and the velocities from the presplit blast measured 685 mm/s.
With this data, assessment of damages was done and comparison of production blasting versus wall control (presplit) blasting were made. First assessed was the PPV. While the above PPV values give general decreasing trend of PPV with each blast, as expected, a plot of PPV versus scaled distance was also done for the production blast and wall controlled blast. While this process is complicated and dependent upon statistical analysis, the results from this showed that the PPV values for the wall controlled blast were lower. However, at distances greater than 60-meters, a convergence of data was observed, signifying at larger distances presplitting has little reduction of PPV values. Summary of the data is shown in Figure 6.2.
Figure 6.2: PPV Data Comparison. Peterson (2001)
Vibration data was then used to calculate properties of the rock mass and these were, in turn, used to estimate fracture criteria based on PPV measurements and tensile strength of the rock. Based on a production blast, the model estimated new fractures with a radius of 5.4-meters, matching well with the observations from the production blast. The pressure data was also used to estimate damage. The underlying principal for this estimation is that an increase in volume will create a decrease in pressure, or as the rocks or rock joints expand, then the pressure measured will drop. This accounts for the wall-control blast and the production blast, but for the presplit blast, a positive pressure was measured indicating gas penetration from the blast. The greatest damage, though, is correlated with the dilation, and therefore the larger blasts performed in the production and wall control blasts.
Recommendations from these observations were given as well. Peterson concluded that vibrations do cause damage, as noted by the new fractures, but when it comes to instability of the final face, it is dilation and heave that cause the most harm at Ekati. The conclusion, then, is that it is not gas penetration that causes damage necessarily, but the movement of the blocks ahead of the gases. Reducing confinement in the larger blasts and distributing the blast energy over greater areas can reduce the subsequent heave associated, increasing stability of the remaining rock mass. Presplitting is beneficial to this end by allowing movement along the face instead of into it, and as noted in the presplit blast discussion, by distributing the charges over many drilled holes. While presplit blasting was beneficial, to improve stability of the final face requires proper design of all the blasts, especially those directly in front of the presplit face.
Comparison of Blast-Induced Damage between Presplit and Smooth Blasting of High Rock Slope by Hu et al. (2013)
Advance of computers has allowed for more modeling techniques over the last decade. This is noted by the use of numerical modeling comparing the damages of presplit and smooth blasting for the study done by Hu et al. Although both types of damage control can provide satisfactory results, the mechanisms for which the damage occurs from each are different, and therefore preventing the extent of the damage must be done differently. As Hu et al. states, damage is caused by the final face blasting, whichever method is chosen, and then the accumulation of damage from all other blasts.
In determining the damage, the model had to capture the initial compressive damage and then the ensuing tension damage. This was done by combining the two damages through mathematical relations and then providing them simultaneously in the model. Next, a system was implemented to capture the damage accumulation from each step. To do this, the stresses and strains from the previous step must be remembered by the elements for the next step while the rock section is removed, replicating conditions for an actual blast. Parameters for the explosives were found, as well as the attenuation rate of the rock. For the rock parameters, data from the Xiluodu Dam high rock slope was used, and similar to the Ekati mine, a plot of the measured PPV vs. scaled distance was created. This was compared to the model constructed PPV values. The above explanation is a very simplified process as to what was actually done for setting up the model, but for the purpose of the paper, focus will be placed more on the results.
With the models set up, the smooth blasting technique was looked at first. One should expect from the sequence of detonation that there will be little reduction in the cumulative damage from smooth blasting because it is detonated last. Looking at the model data, this proved true. Figure 6.3 taken from Hu et al. shows this with captures of each sequence of the blasting process. In the first production blast, the damage extends into the remaining rock mass and then, the second production blast adds to this with a much greater degree of damage. The buffer blasts do not change the amount of damage noticeably when compared to the second production blast, but a noticeable change of damage along the face is induced from the smooth blast. Noticeable damage from the buffer blasts is not seen due to the reduction of explosives and the buffers still being at great enough of a distance from the face. On the other hand, damage is seen from the smooth blast in a column due to the close proximity and geometry of the blast. From analyzing points in the mesh, it was concluded that damage to the main rock mass was a result of production blasting while the column directly surrounding the smooth blasts saw the greatest amount of damage from the smooth blast itself. Damage from buffer blasting had the least effect on overall damage.
Figure 6.3: Damage Process for Each Step of Excavation in Smooth Blasting. Hu et al. (2013)
Presplitting was then analyzed, and in contrast to smooth blasting, cumulative damage should be affected by the presplit blast because it is first in the blast sequence. Figure 6.4 from Hu et al. shows the reduction of damage spreading to the main mass from the presplit. The initial presplit blast causes damage along the final face in a column, but this does not extend into the main rock mass as the first production blast did in smooth blasting. Then, when the production blasts are fired, the extent of damage is halted at the presplit contour. However, when comparing the zones around the final face, the extent of columnar damage around the presplit face, noted by the red in Sequence I, is about 30% higher than that of the smooth blast.
Figure 6.4: Damage Process for Each Step of Excavation in Presplit Blasting. Hu et al. (2013)
The benefits and downsides for each technique must be taken into consideration when designing for a blast. The benefit presplitting provides by reducing the damage to the main rock mass from production blasting should be taken advantage of, but the 30% increase of damage around the presplit face should attempted to be reduced. To accomplish this, Hu et al. suggests a modified presplit where the first production blast is detonated, next the presplit, and then, the final production blast and the buffer holes. This has the supposed effect of limiting the much greater damage seen in the second production blast of smooth blasting while reducing the overburden in the presplit blast, and thus the confinement and ensuing columnar damage.
In comparing the High Rock Slopes study to the Ekati Mine study, it is interesting to note that for the high rock slopes no gas penetration sensors were used to collect data. This was because the objective of the paper was to compare smooth blasting with presplit blasting, but if a similar study were done incorporating gas pressures as well as vibration data into a numerical model, the results for the high rock slopes may have resembled those closer to the Ekati mine, where block heave was the dominating blast damage mechanism. However, the two geologic conditions of the two sites were also different and most definitely affected the studies to a degree and would need to be accounted for when comparing.
Conclusion
The use of explosives to remove rock from unwanted areas will continue to be used, not only in surface blasting, but for underground and underwater blasting as well, because the method is so effective. However, reducing damage to the final rock mass is important to ensuring safety and allowing for more economical designs. The effect damage has on strength in design can be easily seen in the Hoek-Brown equation by the disturbance factor, D, used specifically for damage to a rock mass, both for mechanical, good blasting, and poor blasting excavation (Hoek 2007). Determining the extent of this damage to more refined levels has helped pinpoint the causes of the damage, and in so doing, allowed for designs geared towards remedying the site specific issues. Numerical modeling will prove to be of great use, as it has for the entire field of rock engineering, as long as it is used knowing the limitations of the models. If this is done, however, as it was for the high rock slope projects in China, the sequence of damage is reduced down from a very complicated interactions to a few snapshots of the computer screen that can be interpreted with ease. Rock blasting has advanced from the simple relations passed down through generations of miners into being modeled and researched by engineers working to help improve the safety of said miners. The mixing is a beautiful combination of old and new, seen across all engineering to literally help shape the face of the earth.
References
- Dick, R. A., Fletcher, L. R., and D'Andrea, D. V. (1982). Explosives and Blasting Procedures Manual, Bureau of Mines, Washington, D.C.
- Hoek, E., Carranza-Torres, C., and Corkum, B. "Hoek-Brown Failure Criterion - 2002 Edition". Proc. NARMS-TAC Conference, Toronto, 2002, 1, 267-273.
- Hoek, E. (2007). "Blasting Damage in Rock." Practical Rock Engineering.
- Hu, Y., Lu, W., Chen, M., Yan, P., and Yang, J. (2013). "Comparison of Blast-Induced Damage Between Presplit and Smooth Blasting of High Rock Slope." Rock Mechanics and Rock Engineering, 47 (4), 1307-1320.
- Langefors, U., and Kihlstrӧm, B. (1978). The Modern Technique of Rock Blasting, 3rd Ed., Wiley and Sons Inc., NY.
- Peterson, J. A. (2001). Blast Damage Mechanisms at Ekati(TM) Mine (Order No. MQ69811). Available from ProQuest Dissertations & Theses A&I; ProQuest Dissertations & Theses Full Text; ProQuest Dissertations & Theses Global. (304744467).
- U.S. Army Corps of Engineers (USACE). (1972). Systematic Drilling and Blasting for Surface Excavations Engineering Manual. Engineer Manual 1110-2-3800, U.S. Army Corps of Engineers, Washington, D.C.
 Charles Krolikowski
Charles Krolikowski 





























11 COMMENTS
JH Taljard*
Apr, 07, 2015 Well documented, will review thoughts at a later stage.Excellent idea of making the literature available.
Charles Krolikowski
Apr, 27, 2015 JH Taljard,Thank you for taking the time to read the report and for the compliment. If you have any further thoughts on the report, I will happily accept them.
Thank you,
Charles
Edit Comment
s. figuers*
Apr, 08, 2015 Blasting is difficult field to find information about. A key source of information is the ISEE (international society of explosive engineers). The headquarters are located in cleveland Ohio, and there are local chapters throughout the US. There is one in your area. the monthly publication 'explosive engineering' has good articles. A better source is the proceedings of their annual meetings (generally 1000+ pages long). These are very hard to find. almost none are in any library in the US. However, each chapter has a full set that is available. The chapters have one to several meeting per year and enjoy having non-members.s. figuers*
Apr, 08, 2015 you should review the following:INVESTIGATING THE EXTENT OF DAMAGE
FROM A SINGLE BLASTHOLE- 2014- erickson
a masters thesis - available on line
Charles Krolikowski
Apr, 27, 2015 s. figueres,Thank you for visiting the report site and for the comments. As I was writing the report, I realized that a few of my sources were fairly dated, so the suggestion of looking into the ISEE chapter for future material is much appreciated. This is especially so seeing how close one of the chapters is and now being able to read about current events in blasting through the monthly publication or the meeting proceedings. This was a resource I had not looked into but will take advantage of your suggestion. The thesis you recommended was also very beneficial in providing a more in-depth look at blasting and I will review in greater detail at a later date.
Thank you,
Charles
Edit Comment
John Sturman*
Apr, 08, 2015 I thought that this paper was well-written and the author used illustrations effectively. As a former lecturer in this course and professor (adjunct), I am reluctant to pass to much judgment on a paper without knowing the assignment. But I thought that this paper was cogent and interesting.My concerns related to vibration-related damage are generally much more concerned with adjacent structures than the rock mass itself. Having said that, I think that the author drew from their sources appropriately. Keep going!
Charles Krolikowski
Apr, 28, 2015 John Sturman,Your time reading the report and the comments over it are greatly appreciated. The extent of the report was to simply delve deeper into a topic related to rock mechanics to greater lengths than covered in our class. As such, hearing from professionals and professors actually in the field was also one of the goals of the report, and your judgement is welcomed.
As for the damage to existing structures, initially that was to be included as well, but with limitations on the length, the discussion and case studies related to damage of buildings were omitted.
Thank you,
Charles
Edit Comment
DM Cregger*
Apr, 09, 2015 good overview of an issue applicable to mining and civil engineering; most importantly, backed up by case histories. in the rock damage discussion, the author should indicate at what distance the PPV limits are measured. a discussion of the frequency of the blasting vibrations may be appropriate as shown in many of the newer USBM charts used for control. Charles Krolikowski
Apr, 28, 2015 DM Cregger,Thank you for reading the report and for the comments. Regarding the distance of the PPV measurements, for the Ekati Mine study they were taken 5 meters behind what was considered the final face. As for the table of acceptable PPV measurements and for the high rock slope study, distances were not given in the references.
The importance of the frequency of vibrations could be emphasized more. Due to length restrictions, the report focused more on the actual PPV measurements because those were discussed more in depth for the case studies. However, I had not looked at much of the USBM literature and I appreciate the suggestion regarding the frequencies. The matching of frequencies to joint spacing was briefly mentioned but this could be expanded more.
Thank you,
Charles
Edit Comment
vasiliki*
Apr, 09, 2015 Charles, well done on this report!Having no prior background in blasting, it certainly gave me an valuable insight!
Only a few observations below:
In paragraph 4.4 you refer to bench height and figure 3.3, but there is not such figure in your report.
I would try to better organize sections 5.2 and 5.3, where you explain smooth and pre-split blasting.
In Figure 6.3 it appears that the blasted area is lower than the other three figures. And also the main rock mass does not seem to retain its size between the consecutive blasting phases. Could you please check on this?
The last paragraph of section 6.2 refers to the first case study, which I would prefer to have it incorporated in the previous section.
I also think that mentioning the disturbance factor D, and providing the Hoek – Brown equation earlier in your report would greatly improve its structure. Perhaps looking into how D is affected by the different blasting methods would also make a difference.
Also, I noticed a typo in your conclusions: “However, reducig damage…”
Again, nice work!
Charles Krolikowski
Apr, 28, 2015 Vasiliki,Thank you for taking the time to review the report and to comment. In regards to your comments:
Figure 3.3 in section 4.4 was mislabeled and changed correctly to Figure 4.3.
Section 5.2 and 5.3 were edited and an additional section, 5.4, was added to help with the organization.
As for the figure 6.3, the difference in size of areas is attributed to scaling of the figure, as it should be constant throughout.
At the end of section 6.3, the Ekati Mine was incorporated to compare the two studies and this was edited to signify this.
The Hoek-Brown criterion was added to the beginning of section 5 and includes the disturbance factor D. This was felt to be a more appropriate area to expand on it and tie into the paper.
The spelling error has been corrected.
Again, thank you for your time and glad that the report was insightful.
Thank you,
Charles
Edit Comment